
One of the first questions I ask all of my students is to tell me what they like and dislike about math.
Fractions are almost always the topic that kids say they dislike the most.
Why are fractions so hard for students to understand?
They don’t understand fractions are numbers.
When asked to define a fraction, kids will often say it’s the number of shaded boxes.
To make fractions relatable, pizzas and chocolate bars are used to illustrate fractions. Kids are shown circles (pizzas) or rectangles (chocolate bars) divided into 4 parts and asked would you rather have 2 pieces or 3 pieces? Then they are told the picture with more pieces shaded in is the larger number. This explanation leads kids to believe that they are comparing 2 and 3 boxes - that is, whole numbers.
But that’s not the whole story.
Students need to explore fractions using hands on materials so they can understand that fractions are equal parts of a whole.
They need to work with Fraction Strips and Fraction Circles, Cuisenaire Rods, Pattern Blocks, and Geoboards so they can see that the more parts a whole is divided into, the smaller each fractional part is. (Disclosure: I earn a commission if you purchase any items using the above Amazon links.)
Using these concrete resources, students will discover how fraction equivalents relate to each other.
Students need to compare different sized fractions of the same whole to understand 3 one-fourth pieces (¾) is greater than 2 one-fourth pieces (2/4), but they are both less than one. And 2 one-third pieces (⅔) is more than 2 one-fourth pieces (2/4).
They need to understand that there are fractions in between all whole numbers, not just 0 and 1 by constructing a number line.
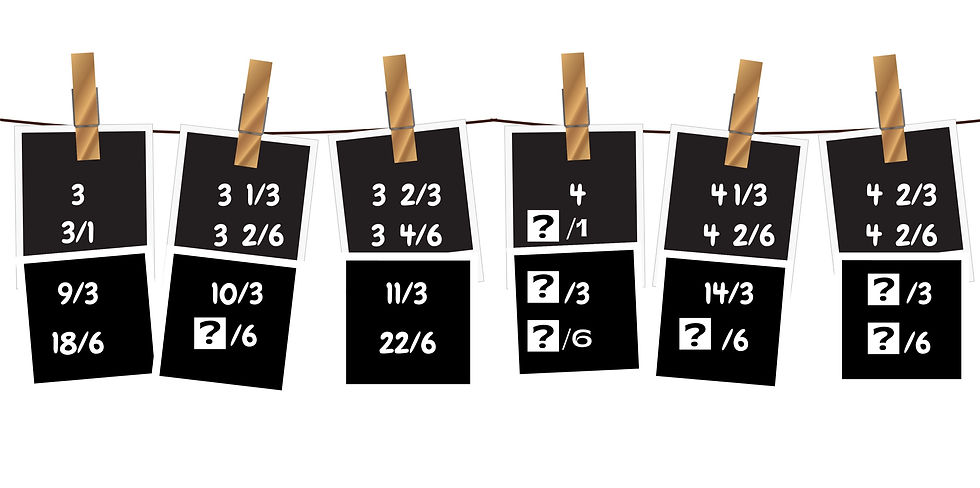
They need to skip count by fractions, not just by 2s, 5s and 10s.
Students need lots of time and many exposures to these concepts before moving on to other fraction skills.
Unfortunately, many students are rushed to fraction operations before they have had the chance to develop strong fractional number sense.
There are different rules depending on the operation.
Adding, subtracting, multiplying, and dividing whole numbers are fairly straightforward. It doesn’t matter if the number is one or more digits - the method is the same.
Not true with fractions. So students are given a set of rules and steps to follow.
Addition or Subtraction: add or subtract the numerator only. First make sure the denominators are the same. If they aren’t, find equivalent fractions with like denominators.

When students have been given the time to make connections and conclusions about fractions, they understand only same sized fractional parts of the same whole can be added together so they know WHY they don’t add the denominators.
Multiplication: multiply the numerator and denominator.

Up to this point in a student’s math journey, multiplication has always resulted in a larger number but when multiplying a fraction this is not the case.
When students have fraction number sense, they understand that they are multiplying a number by a part of another number.
Division: invert the second fraction and multiply.

Many teachers use Keep Change Flip (KCF) to help students remember the steps, but students don’t understand why they are multiplying when they started out with a division equation.
Without a solid understanding of what fractions are and what each operation means, students are bound to get confused...and frustrated.
We are short-changing students and setting them up for failure and frustration when we don’t explain the meaning behind mathematical skills and concepts and just tell them how to do math.
Fractions are essential to daily life -- following recipes, calculating discounts and tips, comparing rates, investing money, etc.
Additionally, fractions are important to the success of upper level mathematics courses.
In 2008 the National Mathematics Advisory Panel published a report, Foundations for Success which concluded that
algebra is the gateway to success in high school and college, and
the main reason for U.S. students’ failure in algebra is their poor proficiency with fractions.
Let’s stop having students memorize rules and procedures. Let's let them make sense of what they are learning and doing.
Who knows? They might not dislike fractions so much if they understand them.

Comments